Processes and reversibility
A process is a change in the system from one state to another. Hence the change in the pressure and temperature of a mass of ideal gas from (p1, v1) to (p2, v2) is a process. The state defined by (p1, v1) is the initial state of the system, and the state defined by (p2, v2) is the final state of the system.
Processes can be divided into two types as follows.
1. Reversible processes these can be reversed so that both the system and the surroundings are returned to their original condition after the process and the reverse process have been carried out.
2. Irreversible processes these are ones in which this reversal cannot be carried out without leaving some change in the system or the surroundings.
The concept of reversibility is a very important one and is best illustrated by a number of examples.
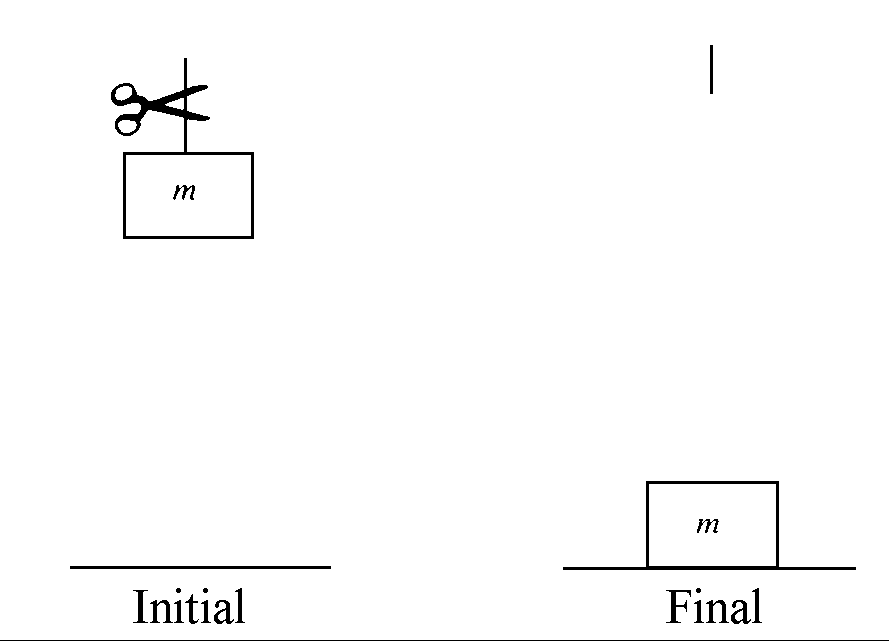
Figure 1 A mass is dropped onto a surface and comes to rest - an example of an irreversible process
1. If a mass is dropped onto a surface (see Figure 1), the mass loses potential energy–a form of mechanical energy. This mechanical energy is lost as the mass comes to rest. A little of it is converted into sound energy which is absorbed by surrounding material and has the effect of heating the absorbing material very slightly. More is converted directly into heat by the action of friction in the material onto which the mass is dropped. This conversion of mechanical energy into heat energy is a sure sign of an irreversible process because as will be proved later, all this heat cannot be converted back into work.
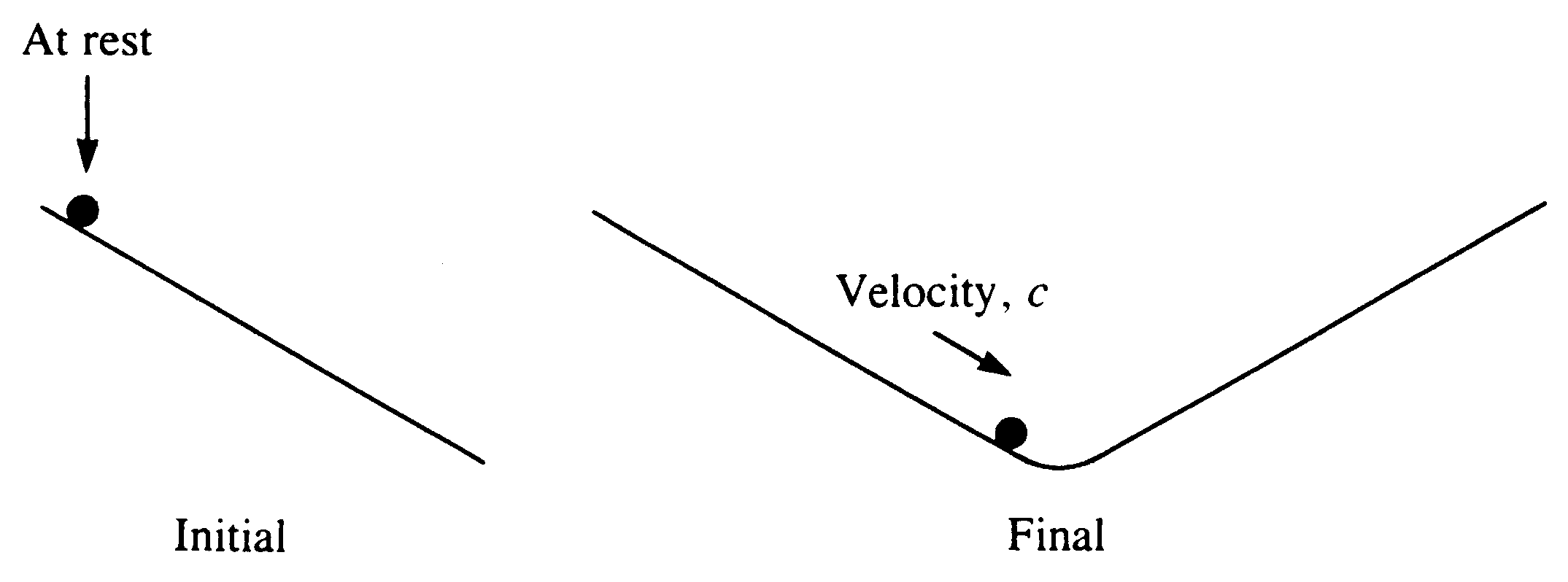
Figure 2 A ball-bearing rolls down a slope–an example of a reversible process
2. If a ball-bearing rolls down a slope without friction the ball loses potential energy, as in the last example. However this time the energy is converted into kinetic energy. This time it is possible to imagine that the process can be reversed, by causing the ball to roll up a similar slope, see Figure 2. In theory and in the absence of friction the kinetic energy will be reconverted into potential energy, and the ball will come to rest at the original height. It is easy to see that any friction will cause the final height to be less than the original height. In general, it is true that friction is a cause of irreversibility in real processes. The potential reversibility of this process occurs because one form of mechanical energy (potential energy) is converted into another form of mechanical energy (kinetic energy).
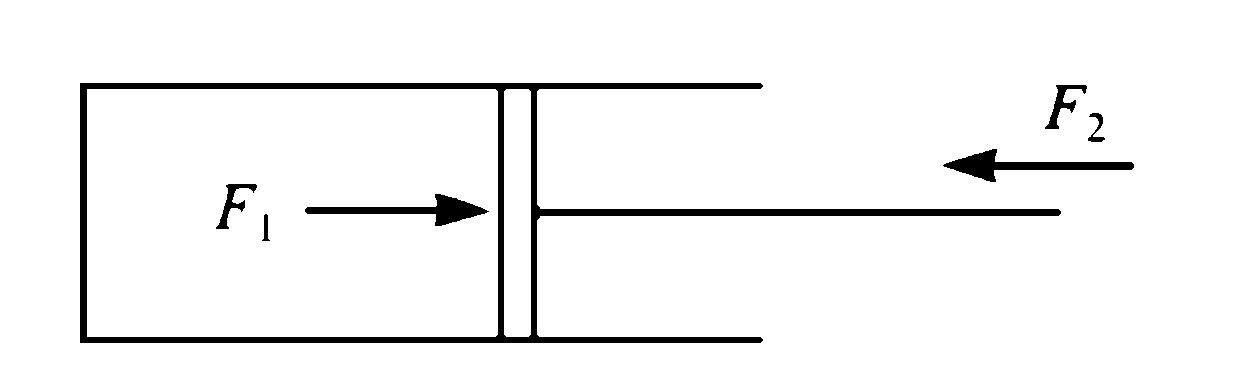
Figure 3 A cylinder containing compressed gas is confined by a movable piston
3. Figure 3 shows compressed gas in a cylinder. A force has to be applied to the piston to keep the gas compressed. Now if the force exerted by the gas on the piston is F1 and the external force applied to the piston is F2, and if F1 is very slightly greater than F2 then the piston will move slowly upwards and the gas will gradually expand. The pressure energy of the gas in the cylinder (a form of mechanical energy) is being converted into another form of mechanical energy as the force F2 is pushed back - perhaps the piston is pushing against a spring. This slow expansion and inter-conversion of mechanical energy is another example of a reversible process. If now F2 is very slightly greater than F1 the gas in the cylinder will be slowly recompressed. Again friction, this time between the piston and the cylinder will tend to cause irreversibility.
4. Alternatively the restraining force on the piston in Figure 3 could be suddenly removed, and the gas in the cylinder allowed to expand quickly. The pressure energy of the gas has decreased, but what has happened to the energy? It has been dissipated in friction at the walls of the cylinder, and in friction between rapidly moving adjacent streams of gas by means of viscous dissipation. The mechanical pressure energy has been turned into heat energy, and so the process is an irreversible one. Expansion of a gas in a cylinder, as in Figure 4 can only be reversible if the expansion is fully resisted, in other words if the forces acting on the cylinder are opposite and almost equal.
Processes can be described in other ways: if a process is carried out at constant pressure it is said to be isobaric, if it at constant temperature it is said to be isothermal, and at constant volume isochoric. Diabatic processes may exchange heat with the surroundings, but adiabatic processes are thermally insulated from the surroundings.

Figure 4 Various types of process on a p-v diagram
Processes can be illustrated as a series of points on a plot of two properties of state. A plot of pressure against specific volume: a p-v diagram is often used for this purpose, see Figure 5 where isobaric, isochoric, and (for an ideal gas) isothermal processes are marked. This latter process has the equation for an ideal gas:
pv = C
from Basic Engineering ThermodynamicsBasic_Engineering_Thermodynamics, by Peter B. Whalley. Reprinted with permission of Oxford University Press
Created with the Personal Edition of HelpNDoc: Full-featured Kindle eBooks generator